Chan group
Nonlinear mode coupling and fluctuation induced transitions
in electromechanical resonators

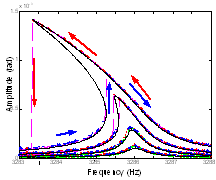

Under sufficiently large periodic drive, nonlinear oscillators develop multistability and exhibit hysteresis in their frequency response. The presence of fluctuations enable the system to occasionally overcome the activation barrier and switch between the coexisting states. Unlike systems in thermal equilbrium, these oscillators are driven far from equilibrium and cannot be characterized by free energy. They possess certain properties that have no analog in equilibrium systems. Apart from fundamental interests, the sharp jump in the oscillation amplitude or phase at a bifurcation point could be used for enhancing detection sensitivity.
Switchings in the driven nonlinear oscillators take place in a two-dimensional phase space. Even though switching occurs randomly in time, in phase space the system follows trajectories concentrated around the "most probable switching path". With our theory collaborators, we demonstrate the breaking of time reversal symmetry in switching of systems far from thermal equilibrium.
We also have observed a number of generic features for switching. For instance, we measured two different scaling of the activation barrier close to the bifurcation points. When the oscillator is resonantly driven into bistability, the activation barrier varies with frequency detuning with critical exponent of 3/2, consistent with predicted universal scaling relationships for saddle node bifurcations. When the oscillator is driven into parametric resonance, the critical exponent becomes 2 as a different kind of bifurcation is involved.
Coupled parametric oscillators: From disorder-induced current to asymmetric Ising model
C. Han, M. Wang, B. Zhang, M. I. Dykman, and H. B. Chan
Phys. Rev. Research 6, 023162(2024). pdf
Energy Transfer into Period-Tripled States in Coupled Electromechanical Modes at Internal Resonance
Yingming Yan, X. Dong, L. Huang, K. Moskovtsev and H. B. Chan
Phys. Rev. X 12, 031003 (2022). pdf
Frequency stabilization and noise-induced spectral narrowing in resonators with zero dispersion
L. Huang, S. M. Soskin, I. A. Khovanov, R. Mannella, K. Ninios and H. B. Chan
Nature Communications 10, 3930 (2019). pdf
Strong negative nonlinear friction from induced two-phonon processes in vibrational systems
X. Dong, M. I. Dykman and H. B. Chan
Nature Communications 9, 3241 (2018). pdf
Correlated anomalous phase diffusion of coupled phononic modes in a sideband-driven resonator
F. Sun, X. Dong, J. Zou, M. I. Dykman and H. B. Chan
Nature Communications 7, 12694 (2016). pdf
Telegraph frequency noise in electromechanical resonators
F. Sun, J. Zou, Z. A. Maizelis and H. B. Chan
Physical Review B 91, 174102 (2015). pdf
Work fluctuations in a nonlinear micromechanical oscillator driven far from thermal equilibrium
P. Zhou, X. Dong, C. Stambaugh and H. B. Chan
Physical Review E 91, 052110 (2015). pdf
Poisson noise induced switching in driven micromechanical resonators
J. Zou, S. Buvaev, M. Dykman and H. B. Chan
Physical Review Letters B 86, 155420 (2012). pdf
Activation barrier scaling for fluctuation induced switching in driven non-linear micromechanical oscillators
H. B. Chan and C. Stambaugh
Journal of Statistical Mechanics: Theory and Experiment, P01028 (2009). pdf
Switching-path distribution in multidimensional systems
H. B. Chan, M. I. Dykman and C. Stambaugh
Physical Review E 78, 051109 (2008). pdf
Paths of fluctuational induced switching
H. B. Chan, M. I. Dykman and C. Stambaugh
Physical Review Letters 100, 130602 (2008). pdf
Activation barrier scaling and crossover for noise-induced switching micromechanical parametric oscillators
H. B. Chan and C. Stambaugh
Physical Review Letters 99, 060601 (2007). pdf
Supernarrow spectral peaks near a kinetic phase transition in a driven, nonlinear micromechanical oscillator
C. Stambaugh and H. B. Chan
Physical Review Letters 97, 110602 (2006). pdf
Fluctuation-enhanced frequency mixing in a nonlinear micromechanical oscillator
H. B. Chan and C. Stambaugh
Physical Review B 73, 224301 (2006). pdf
Noise activated switching in a driven, nonlinear micromechanical oscillator
C. Stambaugh and H. B. Chan
Physical Review B 73, 172302 (2006). pdf